The Tetrahedral World of Washington D.C.

c

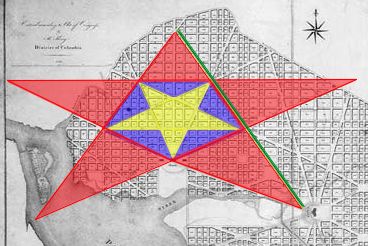
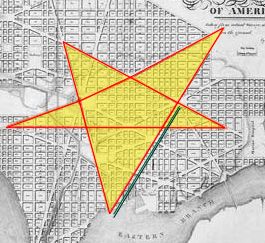
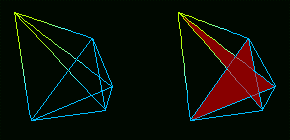
c

Part Four

The Star Spangled City

One thing that has really stood out in Cydonia Quest's investigations of the L'Enfant street plan for Washington DC is the large number of pentagrams in the design in addition to the more familiar White House pentagram discussed in Part Two, (see µµµ). Generally, these Washington pentagrams are distorted and many of them burst the boundaries of L'Enfant's city. They often share components with the tetrahedron images described in Part Two. Indeed, if the pentagrams were properly proportioned these particular tetrahedron images would be incredibly elongated and unconvincing as a design element. The illustration below shows a pentagram to the north of the White House which partially overlaps with the White House Pentagram. It can be seen that its squashed appearance is due to its combination with a tetrahedron image that was shown in Part Two, (µµµ). (Both this particular tetrahedron and pentagram depend on the "missing avenue" that is needed to complete the symmetry of the plan around the 16th Street north/south axis of symmetry, (µµµ).)

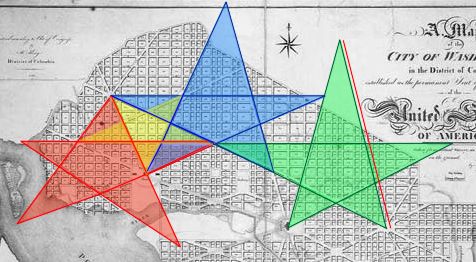
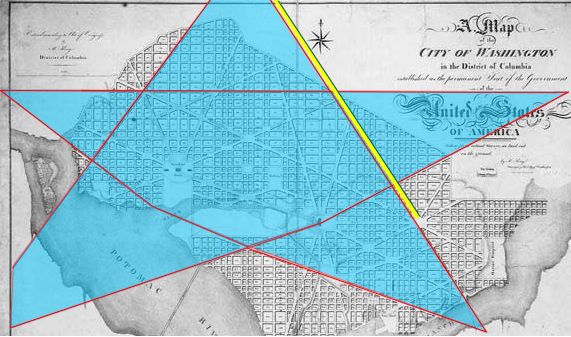
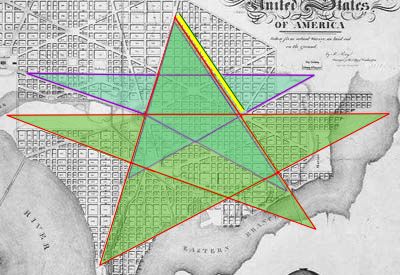
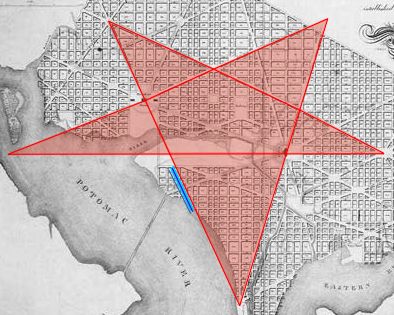
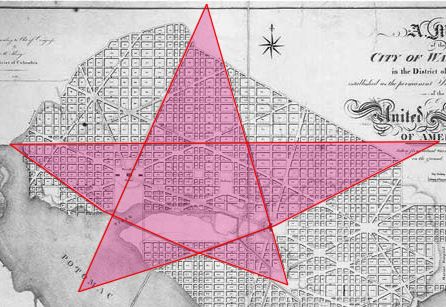
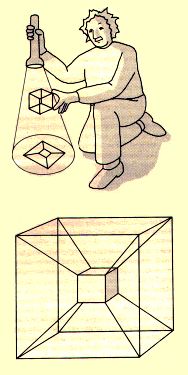
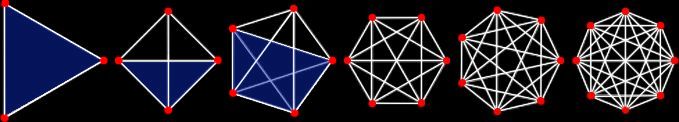
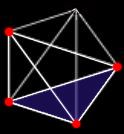
ccccccccccccccccccccccccccccccccccc

c

The White House Pentagram is the only one in the plan surrounded by a pentagon. This is a clue that it is surrounded by an even larger pentagram. In the illustration below the White House Pentagram is shown in yellow. It can be seen that if the lines of the street plan are extended over the city boundary or into the Potomac, and the "missing avenue" (shown by the green line) is added, then a strongly pentagramic pattern emerges.

C

cccccccccccccccccccccccc

C

The next illustration shows an arc of pentagrams formed by the avenues and streets stretching from the west to the east of the city. In the case of the pentagram coloured in green we have to add a line, which is shown in red.

c

ccccccccccccccccc

C

In Part Two it was seen how extending the line of Kentucky Avenue northwards created the boundary for a super tetrahedron image. This same line also completes three large pentagrams. In the first illustration below it can be seen how this extended line (in yellow) creates a super pentagram. Whilst the second illustration reveals a double overlapping pentagram arrangement to the east of the Capitol.

C

Cccccccccc

cccccccccccccccccccccc

C

The counterpart of Kentucky Avenue is Tennessee Avenue. If the line of this avenue (shown in green) is extended southwards then another pentagram overlapping with the two above is completed.

C

ccccccccccccccccccccccccccccccc

C

This review of the pentagrams concludes with two large designs in central Washington. The short blue line shows where one of these cuts across part of the street pattern without there being a corresponding section of avenue. These are not the end of the possible pentagrams that can be created out of L'Enfant's plan, but most of them are covered on this page.

C

ccccccccccccccccccccccc

cccccccccccccccccccc

C

Readers may remember that in Part One it was suggested that -

"......we may be witnessing an insiders' number game. If the angle between Pennsylvania and Maryland Avenues was one degree too great for "perfection" then balance could be maintained by ensuring that the intersection angle between Delaware and New Jersey Avenues was made one degree "too small". Those in on the secret would know that a degree should be taken away from one angle to be added to the other to achieve the desired result."

- when attempting to explain why the planned intersection angle for the north and south beams was narrowed from 33 to 32 degrees, (see µµµ). However, we should also note that the general angle of divergence of 40 degrees for the west beam and the 32 degree angle eventually used for the north and south beams also adds up "fortuitously" to 72 degrees. This is the divergence angle between the points (stellations) in a regular pentagram.

In its public face the pentagram is the most important geometric symbol used in Freemasonry so we should not be surprised that so many of them turn up in L'Enfant's street design. In modern times the pentagram has attracted a sinister reputation as a black magic mechanism through its use by very small fringe groups such as Satanists. This seems to have been the consequence of the opinion by the 19th century occultist writer Eliphas Levi that a downward pointing pentagram was aligned with the infernal forces. (Modern era Western occultists seem to have an obsession with taking millennia old symbols and giving them a dual good or evil meaning in the tradition of the Ying and Yang symbol). In any case the pentagrams of Washington DC are pointing neither up or down. They are pointing either north or south, flat along the ground and L'Enfant would have only have associated good connotations from them. An excellent web page on the history of the pentagram can be read at the end of this "stargate"µµµ.

c

Pentagrams, Tetrahedrons and the 4th Dimension

The three geometrical elements with the greatest preponderance in the L'Enfant plan of Washington DC are the pentagram, the tetrahedron and the hexagram (which probably represents the "star" tetrahedron arrangement). This choice of imagery has a surprisingly hyperdimensional flavour, because the pentagram is the two dimensional shadow of the four dimensional hyper-tetrahedron.

In geometry there are equivalent geometrical entities in every spatial dimension. The cube for example is the 3-D "square". It is impossible for the human mind to comprehend the 4-D hyper-cube equivalent of the 2-D square because there has been no evolutionary reason for us to develop the capability. We just aren't aware of encountering a 4th dimension in everyday life, and although mainstream hyperdimensional physics is now well developed the evidence for the existence of dimensions beyond those of space-time is currently slim.

In his 1910 book The Fourth Dimension the mathematician Charles Howard Hinton developed some visual tricks to help non-mathematicians get to grips with the intricacies of hyperdimensional objects. One of these techniques was to portray the 2-D and 3-D "shadows" of imaginary 4-D objects made out of wire frames. The illustration below is taken from Professor Michio Kaku's book Hyperspace: a scientific odyssey through the 10th dimension. It portrays an Albert Einstein look-a-like creating a shadow of a wire frame cube onto the ground. If a wholly two dimensional creature happened to be passing underneath along the ground at the same time it would be completely perplexed by the changes in the configuration of this 2-D shadow as Mr. Look-a-like rotated the cube or changed the angle from which the torch was shone.

The second figure in the illustration is a representation of the 3-D "shadow" of a 4-D hyper-cube at its most regular. This 3-D shadow comprises of a cube within a cube with all the external edges of the internal cube attached to the internal edges of the outer cube by partitions. This "stargate" µµµ leads to a web page that allows the user to view the shadow of a hyper-cube and to see the results of changing the hyper-cube orientation by using the controls at the bottom of the display. The results are perplexing.

c

cccccccccccccccccccccccccccccccccccc

The original caption with this diagram reads: A Flatlander can visualise a cube by its shadow, which appears as a square within a square. If the cube is rotated , the squares execute motions that appear impossible to a Flatlander. Similarly, the shadow of a hyper-cube is a cube within a cube. If the hyper-cube is rotated in four dimensions, the cubes execute motions that appear impossible to our three dimensional brains.

C

The 3-D "shadow" of a 4th dimension hyper-tetrahedron is called a Pentatope. Although the triangle and the tetrahedron are the simplest geometric shapes in the second and third dimensions, the four dimensional hyper-tetra produces a less understandable 2-D and 3-D shadow than the hyper-cube. The hyper-tetra somehow joins together five regular 3-D tetrahedrons as "facets" on a regular Platonic hyper-solid that can be comfortably circumscribed within a hyper-sphere. (In three dimensions only four tetrahedrons can be fitted onto the facets of another tetrahedron). A variant of a 2-D shadow of a 4-D hyper-tetra is shown in the illustration below. It can be seen that in this particular projection that there is already an irregular pentagram within an irregular pentagon.

C

cccccccccccccccccccccccccccc

C

It is certainly possible to orientate a four dimensional hyper-tetra to produce a regular pentagram. In geometry the simplest geometric figure in each spatial dimension is called the "simplex". The illustration below shows the simplexes from the second to the seventh spatial dimension. The vertices of each simplex as a 3-D object are marked by red dots. The first figure is an equilateral triangle. The second is a tetrahedron seen along its second (and less familiar) type of axis of symmetrical rotation, (see Part Two). The facet of the tetrahedron that would be sitting on the ground if it was a three sided pyramid has been shaded in blue. It can be seen that from this angle that the 2-D shadow of a tetrahedron looks the same as a four sided pyramid seen from above. This is a geometrical link between the tetrahedron and the pyramidion as "Eye of Horus" that was missed in Part Three, so we will note it here because it has some implications for Washington DC later on.

C

ccc

C

The third figure shows the shadow derived from a 4-D hyper-tetra with an equivalent orientation to the tetrahedron that proceeded it. This produces a 2-D shadow that is a regular pentagram. The blue shading and red vertices dots show that the 3-D Pentatope "shadow" is a four sided pyramid with a trapezium shaped base. Interestingly, the five dimensional simplex that comes next in the series has a 2-D shadow that looks exactly like a line diagram for two interlocking tetrahedrons in 3-D.

The reader may be interested to know that the five regular tetrahedrons that make up the "facets" of a hyper-tetra appear as five heavily distorted tetrahedron line drawings within the pentagram. The trick to finding them is to think three dimensionally and to look for them one at a time. The diagram below shows one of these distorted tetrahedrons with the base shaded blue and the four vertices (corners) marked by red dots. In the three dimensional pentatope "shadow" these misshapen tetrahedron overlap with one another and share the same space, which is an impossibility in three dimensions but not in four. For more geometry information on the Pentatope and simplexes try these web pages µµµ & µµµ.

C

Cccccccccccccccccccccccccccccccccccccc

C

If "They" Knew About Hyperdimensional Geometry, Then When did "They" Discover it?

As history tells it proper hyperdimensional geometry began with a lecture given by George Bernhard Riemann in 1854 in which he showed that the geometry of any hyperdimension flowed quite easily and logically from the mathematics of 3-D geometry. However, the argument over the existence of a 4th spatial dimension had been raging since the time of the ancient Greek philosophers (and perhaps even earlier). Greek metaphysicists, such as Plato, argued that the perception that every effect in three dimensions had a three dimensional explanation was an illusion. There was a non-perceivable component involved in all effects as well. This approach was rejected by Plato's pupil Aristotle who seems to have been a great believer in the "common sense" approach to the physical world. Aristotle described the idea of a 4th spatial dimension as "nonsense" and set out the following doctrine.

"The line has magnitude in one way, the plane in two ways, and the solid in three ways, and beyond these there is no other magnitude because the three are all." -Aristotle, from On Heaven

As with many of Aristotle's (often erroneous) opinions this attitude to the possibility of the 4th dimension became the conventional point of view. Of course Aristotle is the authority that kept people thinking that the Sun and planets orbited the Earth for 2,000 years despite the more plausible theories of Heraclides Ponticus, Aristarchus of Samos and Seleucus of Seleuceia that the Earth rotated on its axis and orbited the Sun with the other planets. Much of the credit for saving the Earth centred Universe for so long goes to Ptolemy of Alexandria who tinkered with Aristotle's system so that it would be better at predicting the position of the planets in the sky, (see µµµ). Ptolemy was also the author of an influential treatise that sought to show that four dimensional geometry was mathematically illogical - but only by trying to fit four dimensions into three dimensions which was a methodological flaw.

There seems to have been an unrecorded popular dissidence against this dogma, especially amongst the religious who associated the 4th dimension with the realm of the "unseen", i.e. God, the soul and Heaven. In his Letter to the Ephesians St. Paul raises the mystery of the 4th dimension and fully expects the recipients of the sermon to know what it is he is talking about.

"That Christ may dwell in your hearts by faith: that ye, being rooted and grounded in love, may be able to comprehend with all the saints what is the breadth, and length, and depth, and height; and to know the love of Christ, which passeth knowledge, that ye may be filled with all the fulness of God". -St.Paul Letter to the Ephesians. 3:17-19.

This idea that the "unseen" was the progression of space beyond three dimensions was also taken up in the Middle Ages by St. Bernard. Interestingly St. Bernard was the powerful religious backer of the Knights Templar and a proponent of the use of "sacred geometry" in religious buildings. This would make him a subject of interest to Freemasons.

"What is God? He is length, width, height and depth." -Saint Bernard of Clairvaux, "On Consideration"

An Outrageous Hypothesis. It is now time to put forward a hypothesis for the persistence of the belief in the 4th dimension despite the "informed" rejection of it. (Note: this is a hypothesis and not a claim about what really happened. There is a difference). Suppose that a society of ancient Greek metaphysical philosophers actually attempted to work out the logic of 4-D geometry - or built on earlier attempts in Egypt and Babylonia where mathematics was quite advanced. In the 6th century BC there was such an esoteric society "with secrets" founded by Pythagoras. This mystery school was so influential that it took over the running of the city of Croton in Sicily where it was based.

One of the things about Riemann's proofs of how hyper-dimensional geometry is mathematically logical is that some of them could have been arrived at very easily by Pythagoras if he had the imagination. For example Pythagoras's famous Theorem (which was already known about in Egypt and Babylonia) states that in a right angled triangle the sum of the squared lengths of the smaller sides equals the square of the length of the diagonal longer side (the hypotenuse). The formula for this is written out as A² + B² = C².

This formula is easily uprated to solving the equivalent problem in three dimensions. Suppose we want to know the length of the diagonal between the furthest corners of a right angled object such as a brick. Pythagoras's Theorem can be uprated very easily to say that the square of this diagonal is equal to the sum of the squares of the height, width and length of the brick - or A² + B² + C² = D². If a mystic such as Pythagoras discovered this progression then he would have also realised that finding the length of the diagonal in a 4-D object would be a simple progression to A² + B² + C² + D² =E².

Now to us the proof that 4-D geometry is mathematically logical is not the same as accepting that the 4th dimension exists. We require physical proof as well as abstract proof. This would not have been the case for Pythagoras and his followers or for many other Greek philosophers for that matter. Pythagoras's dictum was that -

"All is number; numbers are the models of things."

This sounds like it describes the role of mathematics in modern science. However, science as we understand it was poorly developed in Pythagoras's time. To the ancients this meant that if something could be shown mathematically then it must also exist in reality. If Pythagoras, or the Egyptians or the Babylonians did extend Pythagoras's Theorem to the 4th dimension and beyond then they would have seen this as proof of the existence of the "unseen" component of reality. (It should be noted that Plato's metaphysics was influenced by the Pythagorean Archytas of Tarentum).

So could the Pythagoreans or the Egyptians or the Babylonians have figured out that the pentagram was the 2-D representation of the 4-D Hyper-tetra. Well, it might answer the mystery of why the Pythagoreans adopted the pentagram as their secret symbol even though it was widely recognised by the general public. (The earliest known pentagrams are found on pottery from the ruins of the Sumerian city of Uruk 5,000 years ago). Maybe it was what the pentagram represented to the Pythagoreans that was the secret.

As was seen in Part Three the tetrahedron was important to the Pythagoreans because not only was it the "atom" for the element of fire but it was also the substance of the soul. The 4-D "supernatural" tetrahedron would have been of great interest to them and they could have described it by simply extending the progression of geometry from the triangle to the tetrahedron still further.

The 2-D triangle has 3 sides.

The 3-D tetrahedron has 4 facets (sides) made out of triangles.

Therefore the 4-D hyper-tetra must have 5 "hyper-facets" (sides) made out of tetrahedrons.

In order to arrive at the pentagram as the 2-D representation of the hyper-tetra they could simply have followed the logic of the line drawing of the tetrahedron shown (again) below. In the diagram of the dimensional progression of the simplexes we saw that in order to see the outlines of all four facets and vertices of a tetrahedron simultaneously, it had to be angled in such a way that its outline appeared as a four sided figure. From this the Pythagoreans could have deduced that the 2-D shadow of a hyper-tetra with its five tetrahedron "facets" must have a five sided outline - a pentagon - within which the five tetrahedron "facets" must be visible. In the case of the tetrahedron diagram the 4 facets and 4 vertices are revealed by drawing lines between the 4 corners of the 4 sided outline. This produces a diagram in which the 4 equilateral triangle facets of the tetrahedron are distorted by foreshortening. From the example of the tetrahedron it would follow that drawing lines between the corners of the 2-D pentagon shadow outline of the hyper-tetra would reveal line drawings of 5 tetrahedrons that had 3-D distortions. This is the actual case as we have seen previously.

C

cc

C

Riemann developed his hyperdimensional geometry because he realised that the field equations for gravity and the electromagnetic force would be simpler and easier to unify if the Universe had more than three spatial dimensions. Unfortunately physics was too under-developed in Riemann's time to make use of his ideas, although Einstein used Riemann's methods to establish time as a 4th dimension in physics. Riemann's work did capture the imagination of late 19th century occultists who, using the analogy of the abilities of a 3-D being in comparison to a 2-D being, were fascinated by the god-like powers a being with access to the 4th dimension would have. They speculated whether it could explain powers of extra sensory perception and mind over matter, (see µµµ).

If some esoteric group such as the Pythagoreans did develop simple 4-D geometry then the same thought would likely have occurred to them. They would probably want to keep 4-D geometry to a select group of initiates for two reasons. Firstly, if a way of utilising the 4th dimension could be found then it would be very dangerous if it was discovered by the wrong people. Secondly (and more selfishly) if the initiates themselves could develop this 4-D "magic" then they would be extremely powerful, so why share the secret?

It is possible that this secret became dispersed across the European and Middle Eastern worlds so that there were initiates in the Jewish, Moslem and Christian religions that knew about it. It is also possible that they have contributed key tetrahedral numbers into religious practices. The Enterprise Mission has drawn attention to an observation made by Rashad Khalifa that the number 19 is heavily encoded into the Koran, (see µµµ). This is pretty close to the tetrahedral angle of 19.5 degrees. (It should be noted that by tradition Mohammed did not directly write or edit the Koran). In Christianity the tradition is that Jesus was on Earth for 33 years, despite New Testament evidence to the contrary. The Enterprise Mission have argued that the number 33 is important to Freemasonry because it is derived from tetrahedral geometry. When we consider the seven axis of symmetrical rotation of a tetrahedron in relation to Christian numbers then the sky is the limit. The number seven has star billing in the Book of Revelations. Other number "7" associated traditions in Christianity include the Seven Deadly Sins, the Seven Passions of Christ and the Fourteen Stations of the Cross. In his work the Inferno Dante gave seven levels each to Hell, Purgatory and Heaven - hence the phrase "in Seventh Heaven".

In regard to the pentagram and Freemasonry some Freemason's today attribute a 4th dimensional significance to this figure. The following "stargate" leads to an article on the website of a Freemason's Lodge that was written about the symbolism of the pentagram by a member of a Rosacrucian Masonic order µµµ. The author asserts that the first stellation of a pentagram represents a geometric point. The second stellation represents a straight line. The third a 2-D plane and the fourth a 3-D solid. The fifth stellation is the expansion beyond three dimensions where the divine can be found. It would not be surprising if Pierre Charles L'Enfant and George Washington saw the pentagram in the same way, uniting man with the supernatural through geometry and the 4th dimension.

In regard to the previous hypothesis it is likely that the Enterprise Mission would comment that it is not "outrageous" enough. There is the question of just why ancient societies with a limited body of knowledge in physics thought that they needed a 4th dimension to explain how the Universe worked or to provide a home for the gods when there was plenty of empty sky to put them in? Readers of the Enterprise Mission will know that they argue that in the pre-historic past human beings have had experience of an advanced technology based on hyper-dimensional physics and that some memory of this has lingered. However, this section is only concerned with showing that Freemasonry may have known in L'Enfant's time that there was a 4th dimensional link between tetrahedrons and pentagrams. There is not the space to consider the Enterprise Mission's idea here.

The Enterprise Mission's Theories on How the Location of Washington DC was Chosen. To finish off this investigation into Tetrahedral Washington some space will be devoted here to the Enterprise Mission's thoughts on the location of the city as set out in their Millennium series of articles.

George Washington was given free rein to choose the location of the new Federal capital. The Enterprise Mission has suggested that there were esoteric as well as practical reasons for the site he chose. They believe that Washington DC was chosen with a specific latitude and a specific longitude in mind.

If one looks at a map of Washington then one will notice that the boundary of the District of Columbia and Arlington County form a square ten miles to a side. The corners of this square are aligned with the points of the compass which means that if the lines of longitude and latitude are drawn from corner to corner the Washington "square" looks like a pyramid from above - or a tetrahedron tipped to show all its vertices and facets. This imagery may not be accidental. In Part Three a description was given of how the ancient Egyptians considered the pyramidion to be a symbol of an enlightening "Eye of Horus" and how L'Enfant may have linked pyramidions with tetrahedrons. Somebody might have intended the Washington "square" to represent a virtual "Eye of Horus".

From the point of view of the Enterprise Mission the interesting thing about this Washington "square" is that its northern tip practically ends at the 39th parallel. This number is of course twice the key tetrahedral number of 19.5 as discussed in Part One. (It was geographically impossible to place the new capitol on either 19.5 degrees longitude or latitude). The reader may remember that 19.5 is a rounding up of the exact tetrahedral latitude of 19.471 degrees (decimal notation). This means that the exact two times harmonic of 38.942 degrees latitude runs through the city.

Atlases give the longitude of Washington DC as either 77 degrees or 77 degrees 1 arc minute. The Enterprise Mission argue that this longitude has an esoteric significance based on some research by Duncan Steele and Simon Cassidy concerning "God's Longitude". The Christian religious calendar set out by the Nicene Council during the Roman Empire combines a number of complicated and illogical rules including using both a solar and lunar cycle. This means that the religious vernal "equinox" does not always correspond with the day of the actual vernal equinox. During the Gregorian calendar reforms a clever system using a 33 year cycle of leap years was proposed that would have matched the religious vernal "equinox" to the actual equinox. The match would have also have been most accurate to the minute at the 77th longitude. Although this system was not adopted, scholarly esoterics knew about it and the special religious nature of a longitude that once again involved the Christian love affair with the number "7". (See µµµ)

If one looks at the institutions whose actions interest the Enterprise Mission (TEM), then they have located themselves in Washington in a way consistent with TEM's theories. NASA's offices within Washington are on Maryland Avenue which as we saw in Parts One & Two plays a role in a scheme consistent with tetrahedral geometry in L'Enfant's plans. On the fringes of Washington, the northern boundary of the grounds of NASA's Goddard Spaceflight centre is delineated by the 39th parallel exactly. Major institutions and property of the Catholic Church form a swathe along the 77th degree longitude as it passes through Washington. This major cluster is centred on where the 77 degree longitude and the tetrahedral 38.942 degree latitude cross. (The following "stargate" leads to a map of the area in which the reader's mouse cursor will give a decimal notation map read-out on the bottom tool bar on the left side µµµ). It could all be just co-incidence, but it is food for thought.

In Conclusion. When this series of web pages began the author only intended to describe a few interesting things in the L'Enfant street plan of Washington. However, one observation led to another and research leads began to suggest themselves. I am therefore surprised how far ranging the articles became as research turned up more and more surprises such as the hyperdimensional link between the tetrahedron and pentagram - or the age old discussion around extra spatial dimensions.

µ Click "Stargate" to Return to Part One

µ Click "Stargate" to Return to Part Two

µ Click "Stargate" to Return to Part Three

c

µ Return to The Conspiracy Latest Developments

µ Click "stargate" to return to The Conspiracy Part Three

µ Return to the Cydonia Quest main page